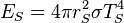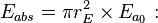http://en.wikipedia.org/wiki/Stefan%E2%80%93Boltzmann_law
鏈子的重點:怎樣計算太陽表麵,地球溫度:
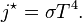

Temperature of the Sun
With his law Stefan also determined the temperature of the Sun's surface. He learned from the data of Charles Soret (1854–1904) that the energy flux density from the Sun is 29 times greater than the energy flux density of a warmed metal lamella. A round lamella was placed at such a distance from the measuring device that it would be seen at the same angle as the Sun. Soret estimated the temperature of the lamella to be approximately 1900 °C to 2000 °C. Stefan surmised that ⅓ of the energy flux from the Sun is absorbed by the Earth's atmosphere, so he took for the correct Sun's energy flux a value 3/2 times greater, namely 29 × 3/2 = 43.5.
Precise measurements of atmospheric absorption were not made until 1888 and 1904. The temperature Stefan obtained was a median value of previous ones, 1950 °C and the absolute thermodynamic one 2200 K. As 2.574 = 43.5, it follows from the law that the temperature of the Sun is 2.57 times greater than the temperature of a lamella, so Stefan got a value of 5430 °C or 5700 K (the modern value is 5778 K[1]). This was the first sensible value for the temperature of the Sun. Before this, values ranging from as low as 1800 °C to as high as 13,000,000 °C were claimed. The lower value of 1800 °C was determined by Claude Servais Mathias Pouillet (1790–1868) in 1838 using the Dulong-Petit law. Pouilet also took just half the value of the Sun's correct energy flux.
Temperature of the Earth
Similarly we can calculate the effective temperature of the Earth TE by equating the energy received from the Sun and the energy radiated by the Earth, under the black-body approximation. The amount of energy, ES, emitted by the Sun is given by:
At Earth, this energy is passing through a sphere with a radius of a0, the distance between the Earth and the Sun, and the energy passing through each square metre of the sphere is given by
The Earth has a radius of rE, and therefore has a cross-section of  . The amount of solar energy absorbed by the Earth is thus given by:
. The amount of solar energy absorbed by the Earth is thus given by:
The amount of energy emitted must equal the amount of energy absorbed, and so:
TE can then be found:
where TS is the temperature of the Sun, rS the radius of the Sun, and a0 is the distance between the Earth and the Sun. This gives an effective temperature of 6°C on the surface of the Earth, assuming that it perfectly absorbs all emission falling on it and has no atmosphere.
The Earth has an albedo of 0.3, meaning that 30% of the solar radiation that hits the planet gets scattered back into space without absorption. The effect of albedo on temperature can be approximated by assuming that the energy absorbed is multiplied by 0.7, but that the planet still radiates as a black body (the latter by definition of effective temperature, which is what we are calculating). This approximation reduces the temperature by a factor of 0.71/4, giving 255 K (−18 °C).[3][4]
However, long-wave radiation from the surface of the earth is partially reflected (or absorbed and re-radiated back down) in the atmosphere, instead of being radiated away, by greenhouse gases, namely water vapor, carbon dioxide and methane.[5][6] Since the emissivity with greenhouse effect (weighted more in the longer wavelengths where the Earth radiates) is reduced more than the absorptivity (weighted more in the shorter wavelengths of the Sun's radiation) is reduced, the equilibrium temperature is higher than the simple black-body calculation estimates. As a result, the Earth's actual average surface temperature is about 288 K (14 °C), which is higher than the 255 K effective temperature, and even higher than the 279 K temperature that a black body would have.